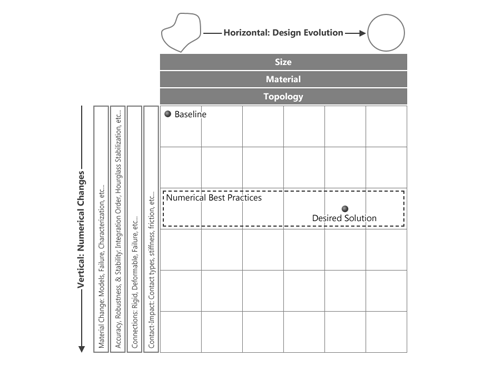
Over the past decade, the ability of simulations driving the design has grown rapidly and today’s confidence in simulations results is a good testament to it. Two significant areas that have contributed to this is the “Numerical Modeling Awareness” and “Design Domain Knowledge” gained over the years by design and analysis community. Numerical modeling awareness is an expertise that is developed over time and goes beyond the ability to learning specific software. It is an awareness in which an analyst, and in essence the corporation, gains expertise in a single or multiple numerical tool set which is used and calibrated against established prototype results to gain more confidence in the modeling procedures and the numerical intricacies involved in a complex software such as LS-DYNA. Design domain knowledge is again an expertise which is gained by a deep understanding of the product and its performance. It’s a knowledge that is more than just knowing how to surface but rather the ability to design a part for a specific criterion in an aggressive timeline yet meeting all the design requirements. In today’s environment, the iterative process quickly fails and mandates a well established standard operating procedure to achieve design requirements. The two areas in a classical LS-DYNA simulation environment that can be grouped together into “Horizontal Changes” (Design Evolution) and “Vertical Changes” (Numerical Changes) are shown below.

(Click image to enlarge)

Horizontal Process
The horizontal process is where the crux of the design changes happen. It could be simple size changes to full-scale topology optimization. An efficient process here is one in which past experiences in product development for load-specific cases are applied as opposed a clean-slate approach. It is here that standard operating procedures and knowledge based engineering can have its biggest impact on product design.

Vertical Process
The vertical process is where a solvers expertise comes into play. Solvers such as LS-DYNA, a generic purpose linear/nonlinear explicit/implicit tool, provides a wide array of features and continues to grow every year to tackle complex problems. Learning to use the software and to make choices in its features to suit a given problem can be daunting at first but can be mastered over time. The vertical numerical process challenges both the developers of the solver, in implementing new features, and its users, in choosing the appropriate features and techniques, to yield most accurate, robust and stable and reliable solution. Several numerical or solver specific modifications can happen in vertical domain which are driven by the motivation to achieve the best possible prediction. Among several potential numerical changes, few examples of changes in this domain include Material models, strain-rate dependency, solver types such as Explicit or Implicit, integration order, Hourglass stabilization procedures, and Contact options. These changes occur either on a frozen design or in some instances could occur over a range of designs. Vertical changes could also involve the choice of modeling techniques such as pure-Largrangian, ALE or SPH which influences the accuracy and scalability of the models. To illustrate this we can consider the modeling of fuels in fueltanks. In a simplistic approach we could use a pure-Largrangian approach which could work when the intent is to use it in small deformations for mass considerations. However, when extending this technique to full-scale sloshing type simulations at high speeds, the limitations of this technique regarding accuracy and scalability will force the analyst to explore other modeling techniques. Fortunately, LS-DYNA provides a range of solution types and in some ways helps the analyst to stay in domain of a single code which dramatically reduces both effort and time when compared to switching to a complete different code to meet the accuracy and scalability requirements. Consequently, a single model for all load cases that can scale well is becoming a reality when solvers offer a wide range of techniques to solve a given problem.